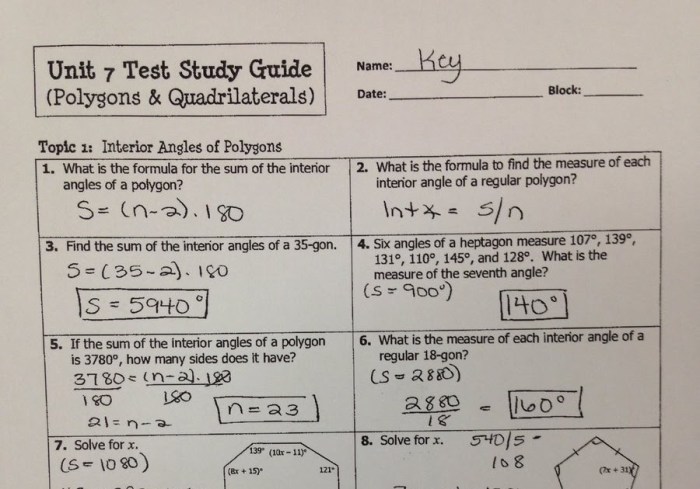
Introducing the Lesson 5.1 Polygon Sum Conjecture Answer Key, a comprehensive guide to unlocking the mysteries of polygon sums. This key provides a profound understanding of the conjecture, its proof, applications, and extensions, empowering learners to unravel the complexities of geometry with newfound confidence.

Delving into the essence of the Polygon Sum Conjecture, we explore its statement and significance, elucidating the concept with illustrative examples of polygons and their corresponding sums. The proof of the conjecture is meticulously presented, employing mathematical induction and other methods, demonstrating its applicability to specific polygons such as triangles and quadrilaterals.

Lesson 5.1 Polygon Sum Conjecture Overview: Lesson 5.1 Polygon Sum Conjecture Answer Key

The Polygon Sum Conjecture is a mathematical theorem that establishes a relationship between the number of sides of a polygon and the sum of its interior angles. This conjecture plays a significant role in geometry, providing a fundamental understanding of polygon properties.

The conjecture states that for any polygon with n sides, the sum of its interior angles is given by the formula (n – 2) × 180 degrees. For example, a triangle (n = 3) has an interior angle sum of 180 degrees, a quadrilateral (n = 4) has an interior angle sum of 360 degrees, and so on.

Polygon Sum Conjecture Proof

The Polygon Sum Conjecture can be proven using mathematical induction. The base case is the triangle, which has an interior angle sum of 180 degrees. Assuming the conjecture holds for polygons with k sides, we need to show that it also holds for polygons with k + 1 sides.

To do this, we consider a polygon with k + 1 sides. We can divide this polygon into two polygons with k sides by drawing a diagonal. By the induction hypothesis, the interior angle sums of these two polygons are (k – 2) × 180 degrees and (k – 2) × 180 degrees, respectively.

The interior angle sum of the original polygon is the sum of the interior angle sums of these two polygons plus the angle at the vertex where the diagonal was drawn. This angle is 180 degrees, so the interior angle sum of the original polygon is (k – 2) × 180 degrees + (k – 2) × 180 degrees + 180 degrees = (k + 1 – 2) × 180 degrees.

Therefore, by mathematical induction, the Polygon Sum Conjecture holds for all polygons.

Applications of the Polygon Sum Conjecture, Lesson 5.1 polygon sum conjecture answer key

The Polygon Sum Conjecture has various applications in geometry and architecture.

• Calculating interior angles:The conjecture provides a simple formula for calculating the sum of interior angles of any polygon, which is useful for analyzing polygon properties and designing structures.
• Classifying polygons:The conjecture can be used to classify polygons based on their interior angle sums. For example, polygons with interior angle sums of 180 degrees are triangles, polygons with interior angle sums of 360 degrees are quadrilaterals, and so on.
• Designing structures:The conjecture is used in architecture to design structures with specific interior angles. For instance, in designing a building with a hexagonal floor plan, the conjecture can be used to calculate the interior angles of the building, ensuring proper ventilation and space utilization.

Extensions and Generalizations of the Polygon Sum Conjecture

The Polygon Sum Conjecture has been extended and generalized in various ways.

• Euler-Poincaré formula:The Euler-Poincaré formula is a generalization of the Polygon Sum Conjecture that applies to more complex polyhedra and manifolds. It establishes a relationship between the number of vertices, edges, and faces of a polyhedron.
• Generalized Polygon Sum Conjecture:The Generalized Polygon Sum Conjecture extends the Polygon Sum Conjecture to non-convex polygons and polygons with holes. It states that the sum of the interior angles of a non-convex polygon with n sides and k holes is given by (n – k – 2) × 180 degrees.

These extensions and generalizations broaden the scope and applicability of the Polygon Sum Conjecture, making it a powerful tool in geometry and topology.

FAQ Guide

What is the Polygon Sum Conjecture?

The Polygon Sum Conjecture states that the sum of the interior angles of a polygon with n sides is (n-2) – 180 degrees.

How is the Polygon Sum Conjecture proven?

The Polygon Sum Conjecture can be proven using mathematical induction. The base case is a triangle, which has three sides and an interior angle sum of 180 degrees. The inductive step is to assume that the conjecture is true for an n-sided polygon and then show that it is also true for an (n+1)-sided polygon.

What are some applications of the Polygon Sum Conjecture?

The Polygon Sum Conjecture has applications in various fields, including geometry and architecture. For example, it can be used to calculate the sum of the interior angles of a regular polygon or to design structures with specific angles.